Scientists Play With Rubber Bands Better Than The Rest Of Us
This article is more than 2 years old
 We’ve all done it: bored at school (probably during math class), you find a rubber band in your desk, or your hair, or even your braces. You start playing with it, twisting it around, seeing what shapes you can make before testing out whether you’re stealthy enough to shoot it at someone without the teacher noticing. When I did this, I didn’t come up with anything publishable in PLOS One, but then again, I wasn’t a Harvard researcher at the time. Those folks found a whole new shape when they played with rubber bands.
We’ve all done it: bored at school (probably during math class), you find a rubber band in your desk, or your hair, or even your braces. You start playing with it, twisting it around, seeing what shapes you can make before testing out whether you’re stealthy enough to shoot it at someone without the teacher noticing. When I did this, I didn’t come up with anything publishable in PLOS One, but then again, I wasn’t a Harvard researcher at the time. Those folks found a whole new shape when they played with rubber bands.
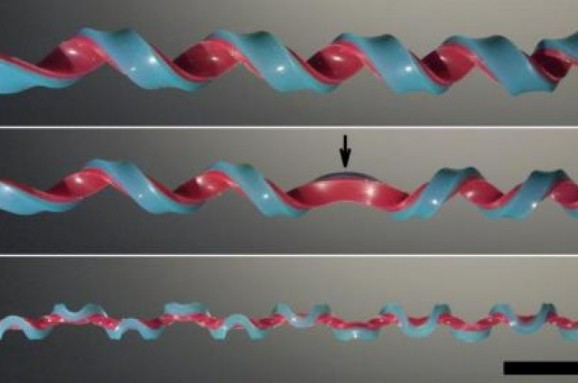
The researchers, who were trying to make cephalopod-inspired springs, glued together two rubber bands of different lengths and then stretched them out using strings, allowing for each of the bands to twist. As they stretched, the strips starting winding, making an unusual shape. Looking at the picture, I swear I remember shapes like this from the days of stretching out the phone cord in an attempt to escape the earshot of my parents. The new shape looks like a double helix, but not quite. It has “perversions” — seriously, that’s the term for it. Yay, science! That basically means that the helix changes direction — it seems at first to curl one way, but then it reverses. Perversions like this exist in nature, particularly in plants that change their direction to angle closer to the sun or to rest on other objects in their pursuit of light. Essentially, what the scientists did was to create something called a “hemihelix.”
The notable difference in this situation is that, when stretched, the rubber bands didn’t demonstrate a single perversion. These were some seriously perverted bands — they changed direction as many as 11 times. The researchers realized that the ratio of the height to width of the cross-section of the band dictated the number of perversions — ones that were wider for their height generated conventional helices, but those with smaller ratios were the ones with perversions.
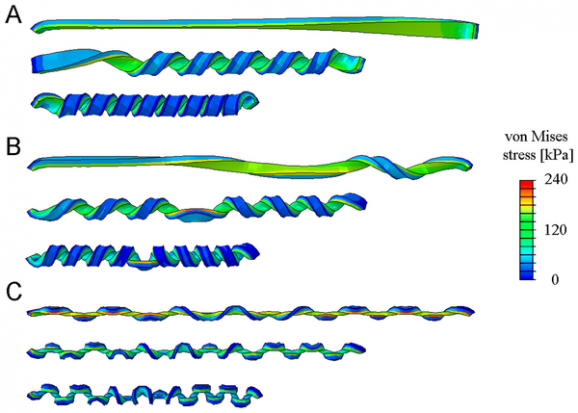
The team believes their finding will allow for the controlling and manufacturing of specific 3-D shapes from 2-D source material. Determining the shapes such strips can make also allows scientists and/or manufacturers to control their resulting properties, such as how they reflect light or, as they point out in their paper, how they can dig into the earth, drill into other materials, or can be used to revolutionize nano-circuitry — that is, so long as the materials can withstand the pressure and don’t snap, which is why this phenomenon likely has been observed before. The cool thing about these shapes is that “there is no randomness…if you make one hundred of these, they’ll always perform exactly the same way,” according to the researchers. The same could be said about me in math class.












